A, B) y=4/5x +11/5
C) x=6
6) Since we have those two points, we can find out the slope between them using the slope formula:
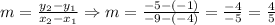
A) In the point-slope form, we can write out the following formula and then plug into that the coordinates the slope found above considering point (-4,-1):

B) The same line described by the slope-intercept formula:
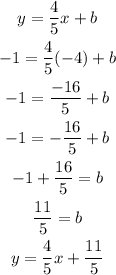
C) Plugging into the function the y-coordinate: 7
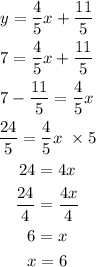
The x-coordinate for y=7 is x= 6.
That's the function y=4/5x +11/5