Question

Write a linear equation for the line that goes through (3, 3) and has a y- intercept of 6. O y = -x + 6 O y=6x+1 O y=-6x-1 O y = 3x + 3

Answer 1

`y=-x+6`

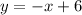
Explanation

we can write the equation in the form

`y=mx+b`

where m is the slope and b is the y-intercept

Step 1

find the coordinate of the y-intercept

when, the line intersects the y axis, the x value is zero, so

`\begin{gathered} \text{coordinate of the y-intercept} \\ (0,6) \end{gathered}`

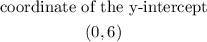
Step 2

find the slope of the line:

when you know 2 points of a line, you can find the slope by using:

`\begin{gathered} \text{slope}=\frac{change\text{ in y }}{\text{change in x}}=(y_2-y_1)/(x_2-x_1) \\ \text{where} \\ P1(x_1,y_1) \\ P2(x_2,y_2) \end{gathered}`

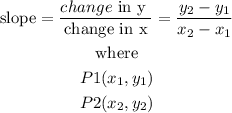
then, Let

P1(3.3)

P2(0,6)

replace,

`\begin{gathered} \text{slope}=\frac{change\text{ in y }}{\text{change in x}}=(y_2-y_1)/(x_2-x_1) \\ \text{slope}=(6-3)/(0-3)=(3)/(-3)=-1 \end{gathered}`

Step 2

hence, we have

slope=-1

y-intercept =6

replace

`\begin{gathered} y=mx+b\rightarrow y=-1x+6 \\ y=-x+6 \end{gathered}`

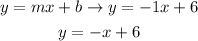
I hope this helps you