Step-by-step explanation:
let the amount of solution used for the 20% concentration = x
let the amount of solution used for the 50% concentration = y
We want to obtain a mixture of 105 liters
amount for 20% + amount for the 50% = 105 liters
x + y = 105 ....(1)
The concentration for the mixture = 22%
20% (amount used) + 50% (amount used) = 105 (22% acid solution)
0.20(x) + 0.50 (y) = 105(0.22)
0.2x + 0.5y = 23.1 ....(2)
Using substitution method:
From equation 1, we can make x the subject of formula
x = 105 - y
we will substitute for x in equation (2):
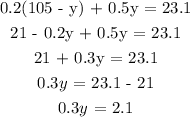

substitute for y in equation (1):
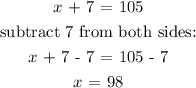
Hence, 98 liters of 20% solution and 7 liters of 50% solution should be mixed