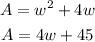
To solve the given system of equations:
1. Substitute the A by the value given in the other equation (equal the equations):
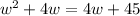
2. Leave in one side of the equation those terms with the variable w and solve it:
![\begin{gathered} w^2+4w-4w=45 \\ w^2=45 \\ w=\sqrt[]{45} \\ \\ w_1\approx6.70 \\ w_2\approx-6.70 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/1x3mahdca2gyoika2oc1dlcg60ektzmo8k.png)
Then, the system of equations have two solutions.
Since w is the width of a rectangle it cannot be a negative value. Just one of the solutions is viable.
There are two solutions, but only one is viable