Answer:
Given that,
Rectangle is removed from a right triangle to create the shaded region
To find the area of the shaded region.
Area of the shaded region is
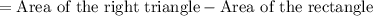
Base of the right triangle is 8 ft
Height of the right triangle is 9 ft
Area of the right triangle is,

Area of the rectangle is,
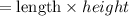
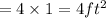
Area of the shaded region is,

Area of the shaded region is 32 ft^2.