Answer:
Step 1: x²(8x + 5)(8x - 5) = 0
Step 2: x = 0, -5/8, 5/8
Step-by-step explanation:
The initial expression is:
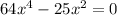
First, we can factorize x², so we can rewrite the expression as:
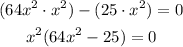
Now, (64x² - 25) is a difference of squares because 64x² = (8x)² and 25 = 5². Therefore, they can be factorized as:
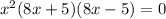
Therefore, the completely factored equation is:
x²(8x + 5)(8x - 5) = 0
Then, this equation is equal to 0 if at least one of the factors is 0, so the solutions of the equation are:
x² = 0
x = 0
8x + 5 = 0
8x + 5 - 5 = 0 - 5
8x = -5
8x/8 = -5/8
x = -5/8
8x - 5 = 0
8x - 5 + 5 = 0 + 5
8x = 5
8x/8 = 5/8
x = 5/8
So, the solutions of the equation are:
x = 0, -5/8, 5/8