Answer:
The ratio of 1.3 to 32 is the same as the ratio of 3.9 to 96
Step-by-step explanation:
The ratio of 1.3 to 32 is
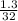
which must be the same as
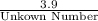
Therefore, we have

Now, cross multiplication gives
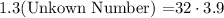
Finally, dividing both sides by 1.3 gives
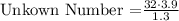
which simplifies to give
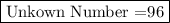
which is our answer!