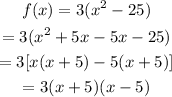Question

Can you help me please? Can you also copy the photo and put the answers in the boxes

Answer 1

Factor the function f(x).

`\begin{gathered} f(x)=3x^2-75 \\ =3(x^2-25) \\ =3(x^2-0\cdot x-25) \end{gathered}`

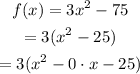
The coefficient of square term is 1, so a = 1.

The coefficient of linear term is 0, so b = 0.

The constant is -25, so c = -25.

The product of a and c is,

`a* c=-25`

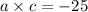
The sum of factor pair must be equal to 0. So factors whoise sum is 0 and product is -25x^2.

`\begin{gathered} f(x)=3(x^2-25) \\ =3(x^2+5x-5x-25) \\ =3\lbrack x(x+5)-5(x+5)\rbrack \\ =3(x+5)(x-5) \end{gathered}`