Answer:
(a)f(x), Common Ratio = 1/4
(b)-12
Explanation:
Part A
From the functions on the table, the function that represents an exponential function is f(x).
The common ratio of f(x) is derived below:
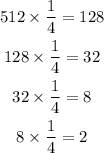
The common ratio is 1/4.
Part B
We want to find the average rate of change for the function h(x) over the interval 2 ≤ x ≤ 4.
From the table:
• When x=4, h(x)=9: h(4) = 9
,
• When x=2, h(x)=33: h(2) = 33
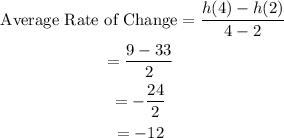
The average rate of change for h(x) over the interval 2 ≤ x ≤ 4 is -12.