Question

Given A be busy prove

Answer 1

We can solve this question if we have that the sum of the interior angles of a triangle is 180 degrees.

We have:

Statement-------------------------------------Reasons

The sum of the interior angles of a triangle is equal to 180 degrees. Then, we have:

`m\angle A+m\angle B+m\angle C=180`

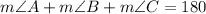
Then, we have that m∠B = 90° (since AB is perpendicular to BC). Then, we have:

`m\angle A+90^(\circ)+m\angle C=180`

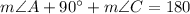
Subtracting 90° from both sides of the equation, we have:

`m\angle A+90^(\circ)-90^(\circ)+m\angle C=180^(\circ)-90^(\circ)`

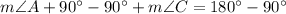
Finally

`m\angle A+m\angle C=90^(\circ)`

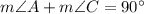
Therefore,

[We have from your homework: "If two angles form a right angle (90°), then they are complementary.]