Answer:
y = (5/3)x + 4
Step-by-step explanation:
To put the equation into the slope-intercept form, we need to solve the equation for y.
So, adding 5x to both sides of the equation:
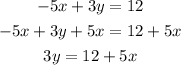
Dividing both sides by 3:
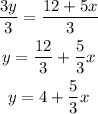
Finally, the equation into the slope-intercept form is:
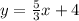
Where 5/3 is the slope and 4 is the intercept.