Question

A surveyor standing some distance from a mountain, measures the angle of elevation from the ground to the top of the mountain to be 69 50 56The surveyor then walks forward 689 feet and measures the angle of elevation to be 79°51'51'. What is the height of the mountain? Round yoursolution to the nearest whole foot.

Answer 1

Given:

The sketch shows the description of the surveyor

The angle of elevation is given in degrees, minutes, and seconds.

Converting this to degrees only,

`\begin{gathered} 69^050^(\prime)56^(\doubleprime)=69+(50)/(60)+(56)/(3600)=69.849^0 \\ \\ \text{Also,} \\ 79^051^(\prime)51^(\doubleprime)=79+(51)/(60)+(51)/(3600)=79.864^0 \end{gathered}`

Representing the sketch as a line diagram,

This line diagram can further be represented by two right triangles;

Using the trigonometric identity of tangent to get the height (h) in both right triangles;

`\tan \theta=\frac{\text{opposite}}{adjacent}`

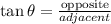
Hence, from triangle A,

`\begin{gathered} \tan 69.849=(h)/(689+x) \\ 2.7251=(h)/(689+x) \\ \text{Cross multiplying,} \\ h=2.7251(689+x) \\ h=1877.5939+2.7251x \end{gathered}`

Also, from triangle B,

`\begin{gathered} \tan 79.864=(h)/(x) \\ 5.5936=(h)/(x) \\ \text{Cross multiplying,} \\ h=5.5936x \end{gathered}`

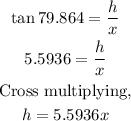
Hence, equating the height (h) gotten in both triangles,

`\begin{gathered} 1877.5939+2.7251x=5.5936x \\ \text{Collecting the like terms,} \\ 1877.5939=5.5936x-2.7251x \\ 1877.5939=2.8685x \\ \text{Dividing both sides by 2.8685,} \\ (1877.5939)/(2.8685)=x \\ x=654.556ft \end{gathered}`

To get the height of the mountain; recall from triangle B,

`\begin{gathered} h=5.5936x \\ h=5.5936*654.556 \\ h=3661.32 \\ \\ To\text{ the nearest whole foot,} \\ h=3661ft \end{gathered}`

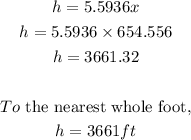
Therefore, the height of the mountain to the nearest whole foot is 3661 foot