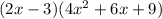The given expression is
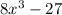
To find the equivalent expression, we use the formula for the difference of perfect cubes.
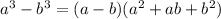
But, we have to express the numbers 8 and 27 as cubic powers.
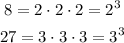
Then, we know that a and b are
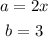
So, the difference would be
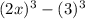
Once we have the difference expressed in cubes, we apply the formula using the a and b values we determined before.
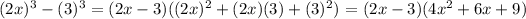
Therefore, the factors are