
Here, we want to get the maximum number of gigabytes
We have this as follows;
The fixed cost (flat cost) is $60.50 per month
The variable cost per gigabyte is $3 per gigabyte
Now, he wants to keep the bill under $95; that means the cost cannot exceed $95
Let the number of gigabytes be x; at $3 per gigabyte; the total is ;
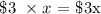
Adding this to the flat cost;
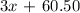
Both of this is less than $95
Thus, we have;
