Let's say the two brands are Brand A and Brand B.
Brand A: 5% vinegar
Brand B: 15% vinegar
X = amount of Brand A
Y = amount of Brand B
Goal: 230 milliliters of 9% vinegar
See the illustration below.
From the illustration above, we can get the following equations:
First Equation: X + Y = 230 mL
Second Equation: 0.05x + 0.15y = 20.7
(Note: 20.7 here is .09 multiplied to 230)
Now that we have a system of linear equations here, we can now solve for the X and Y (amount of each brand). We can do substitution, elimination, or matrix to solve this. But for this equation, let's use substitution.
Let's equate the first equation into Y:
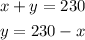
We will substitute y = 230 - x to the second equation.
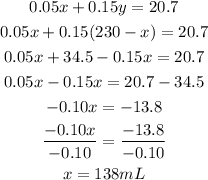
Now that we have the value of x, let's solve the value of y. Substitute the value of x to the first equation.

Therefore, 138 mL of 5% vinegar and 92 mL of 15% vinegar must be combined in order to produce a 230 mL of dressing that is 9% vinegar.