Question

Hello please use original message to do the work and to have the final explanation.

Answer 1

we are asked to determine the sine function of the given graph. To do that we will use the general form of the sine function:

`y=A\sin (k(\theta-b))+c`

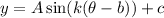
"A" is the amplitude and is the maximum value of the function in this case the maximum value is 6, therefore, A = 6.

The value of "c" is the upward shift of the graph, in this case, the graph was an upward shift of 3, therefore, c = 3}

The value of "k" can be found from the period using the following formula:

`T=(2\pi)/(k)`

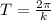
The period, in this case, is 4, replacing in the previous equation:

`4=(2\pi)/(k)`

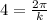
Solving for "k":

`k=(\pi)/(2)`

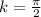
The value of "b" is the horizontal shift of the graph, in this case is: