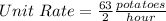Given that:
- Amber can peel this amount of potatoes:
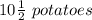
- And she can peel them in this time:

You can determine that the Unit Rate is the number of potatoes she can peel per hour.
Since the number of potatoes is given as a Mixed Number, you can convert it to an Improper Fraction as follows:
1. Multiply the Whole Number part by the denominator.
2. Add the Product to the numerator.
3. The denominator does not change.
Then:
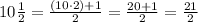
Now you can set up that:
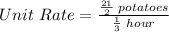
Solving the Division, you get:
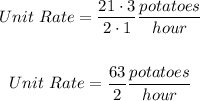
Hence, the answer is: