Question

In the following diagram line C Intersects line D.ABDSy-29282625 4x+53y+193x+7Figure may not be drawn to scale.A. Using complete sentences, classify the relationship between <7 and <8 created by the Intersection of lines C andD.B. Use complete sentences to explain how the special angles created by the Intersection of lines C and D can be usedto solve for y.C. Solve for y and find the measures of <7 and <8.

Answer 1

Part A:

Vertical Angle Theorem states that two opposite vertical angles formed when two lines intersect each other are congruent to each other.

∠7 and ∠8 are a pair of vertical angles. Therefore, they are congruent.

Part B:

By vertical angle theorem, the adjacent angles to ∠7 and ∠8 are also congruent. Equate the two unknown angles, so that we can solve for y.

Part C:

Solving for y, we have the following:

`\begin{gathered} 5y-29=3y+19 \\ 5y-3y=19+29 \\ 2y=48 \\ (2y)/(2)=(48)/(2) \\ y=24 \end{gathered}`

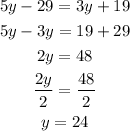
Since ∠7 or ∠8 are linear pairs with (5y - 29) and (3y + 19), they are suppementary which means that

`\begin{gathered} ∠7+(3y+19)=180° \\ \\ \text{Substitute }y=24 \\ ∠7+3(24)°+19°=180° \\ ∠7+72°+19°=180° \\ ∠7+91°=180° \\ ∠7=180°-91 \\ ∠7=89° \\ \\ \text{Since} \\ ∠7=∠8 \\ \text{Then} \\ ∠8=89° \end{gathered}`

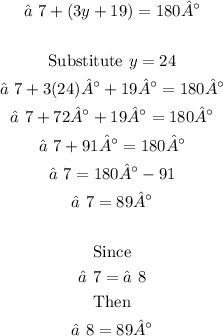
Therefore, the measures of ∠7 and ∠8 is 89°.