Question

The height of an 8th grader is modeled using the normal distribution shown below. The mean of the distribution is 69.1 in and the standard deviation is 2.1 in. In the figure, V is a number along the axis and is under the highest part of the curve. And, U and W are numbers along the axis that are each the same distance away from V. Use the empirical rule to choose the best value for the percentage of the area under the curve that is shaded, and find the values of U, V, and W.

Answer 1

Given

The mean of the distribution is 69.1 in and the standard deviation is 2.1 in.

In the figure, V is a number along the axis and is under the highest part of the curve.

And, U and W are numbers along the axis that are each the same distance away from V.

To find the values of U, V, and W using the empirical rule and the percentage of the area under the curve that is shaded.

Step-by-step explanation:

​It is given that,

The mean of the distribution is 69.1 in and the standard deviation is 2.1 in.

That implies,

`\begin{gathered} \mu=69.1 \\ \sigma=2.1 \end{gathered}`

Since V is a number along the axis and is under the highest part of the curve.

Then,

`V=69.1`

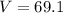
Also, by using empirical rule,

`\begin{gathered} U=V-\sigma \\ W=V+\sigma \end{gathered}`

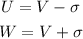
Then,

`\begin{gathered} U=69.1-2.1 \\ =67 \\ W=69.1+2.1 \\ =71.2 \end{gathered}`

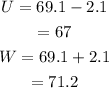
Hence, the percentage of the area under the curve that is shaded is, 68% and the values of U, V, W are 67, 69.1, 71.2 respectively.