There is no intersection between f(x) and g(x)
1) Considering that f(x) is described as:

2) So if we want to check whether both functions intersect each other. Let's examine the table for g(x) function.
If they intersect they must share the same results so let's equate them. But first, let's find g(x)
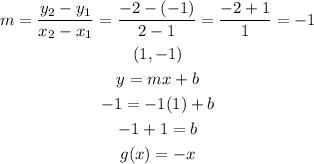
3) Plotting both graphs, we can tell whether there is or there is not an intersection between both functions.
So they do not intersect.