In trigonometry, we use several identities that help us simplify expressions. One of these is:
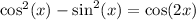
If we look closely, and replace x by the argument of our question, we will see that the expression from our question is already on this form, so we can use the identity above to rewrite the expression.
![\begin{gathered} \cos ^2((5\pi)/(12))-\sin ^2((5\pi)/(12)) \\ \cos (2\cdot(5\pi)/(12)) \\ \cos ((5\pi)/(6))=-\frac{\sqrt[]{3}}{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/lg56zxrruj5fy85w1idk9mvdlrpi7j8k03.png)
The value of the expression is equal to -(sqrt(3))/2.