Question

Can you please help me with this question. Question E the daycare center receives federal funding based on the number of children receiving a subsidy. the amount of federal funding for the current term can be calculated using the formula below. calculate the center’s federal funding for the current term. funding = 1530f + 1750p + 1875n where f = number of children receiving a full subsidy p = number of children receiving a partial subsidy n = number of children receiving no subsidy

Answer 1

Step-by-step explanation

The percentage of 5 years old that received a full or partial subsidy is given by the following expression:

a.

`\text{Full or }partial\text{ subsidy=}(49+11)/(78)=(60)/(78)=0.77`

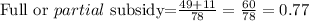
b.

No-subsidy:

`No\text{ subsidy=}(18)/(49+11+18)=(18)/(78)=0.23`

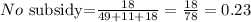
c. 4 years old Full subsidy:

`4\text{ years old full subsidy percentage=}(22)/(26+22+49)=(22)/(97)=0.23`

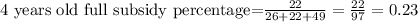
d. Percentage of children that are 5 years old:

`=(49+11+18)/(26+40+38+22+38+13+49+11+18)=(78)/(255)=0.3`

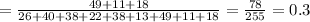
e.

Since the expression of funding is:

Funding = 1530f + 1750p + 1875n

Number of children receiving full subsidy f =

`=(26+22+49)/(26+22+49+40+38+11+38+13+18)=(97)/(255)=0.38`

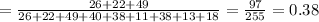
Number of children receiving partial subsidy=

`=(40+38+11)/(26+22+49+40+38+11+38+13+18)=(89)/(255)=0.35`

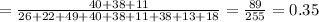
Number of children receiving no subsidy:

`=(38+13+18)/(26+22+49+40+38+11+38+13+18)=(69)/(255)=0.27`

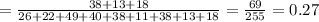
Thus, f=0.38, p=0.35 and n=0.27

Plugging in into the equation:

`=1530\cdot0.38+1750\cdot0.35+1875\cdot0.27=581.4+612.5+506.25=1700`

In conclusion, the center's federal funding was of $1,700