We have a figure FLP with certain coordinates, and we need to reflect these coordinates acroos the x-axis. To better understand this transformation, let's draw a coordinate plane with one point and reflect it across the x-axis, this is done below:
The red dot is the reflection of the black dot across the x-axis. The main concern we need to have is that the distance from the original point to the x-axis must be equal to the distance of the reflected point to the x-axis, but on the other side.
This means that whenever we perform this type of reflection the coordinates of the reflected point will be (x, -y). We only need to invert the signal of the "y" coordinate.
With this in mind we can solve the problem:
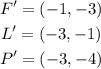
The green dots are the original points, and the red ones are the reflected points.