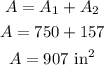Question

Find area of figure. Round to the nearest tenth. 30 in 20 in 45 in (Use 3.14 for i.)

Answer 1

In order to find the area of this figure, let's first find the area of the trapezoid section, where the bases are 45 in and 30 in, and the height is 20 in:

`\begin{gathered} A_1=((B+b)\cdot h)/(2) \\ A_1=(\mleft(45+30\mright)\cdot20)/(2)_{} \\ A_1=75\cdot10=750\text{ in}^2 \end{gathered}`

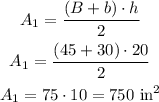
Now we need to find the area of the semicircle.

The radius of this semicircle is half the height of the trapezoid, so we have:

`r=(h)/(2)=(20)/(2)=10\text{ in}`

Calculating the area of the semicircle, we have:

`\begin{gathered} A_2=(\pi\cdot r^2)/(2) \\ A_2=(\pi\cdot10^2)/(2) \\ A_2=50\pi=157\text{ in}^2 \end{gathered}`

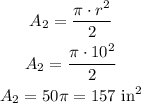
The total area is the sum of the trapezoid area and the semicircle area, so we have that:

`\begin{gathered} A=A_1+A_2 \\ A=750+157 \\ A=907\text{ in}^2 \end{gathered}`