Hello!
First, let's write some important information contained in the exercise:
Fees:
• $8.00 for children
,
• $11.50 for adults
• On a certain day, ,321 people entered to park
,
• The admission fees ,collected totaled $3,173.50
Knowing that we can write it as a system, look:
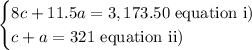
Let me explain the system:
Equation i) means that the total value obtained from the sale of tickets was $3,173.50.
Equation ii) means that the total of adults and children was 321 people.
Okay, now let's solve this system:
I will isolate the variable c in equation ii), look:
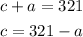
So from now on, we're going to use C = 321 - a.
Now, let's replace the value of C in the equation i):
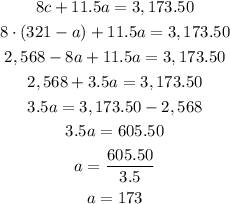
Now we know the number of adults as 173, let's replace it instead A in equation ii):
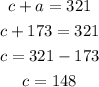
According to the reasoning above, 148 children and 173 adults were admitted.