To estimate the sloep of the tangent line we first need to find an expression for the estimation, we know that this can be done by:
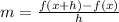
Then we have:
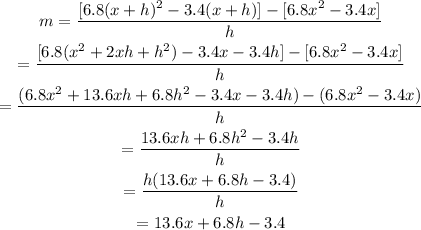
hence the estimation of the slope tangent line is:
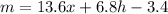
To determine the slope at the given point for the value of h we just plug the values in the expression we found. for example for the first estimate we have:
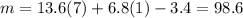
doing this with all the other values of h we have:
h=1: m=98.6
h=0.5: m=95.2
h=0.1: m=92.48
h=0.01: m=91.868
h=0.001: m=91.8068