Question

Can u help me change this repeating decimal to a fraction?The number is 0.210

Answer 1

To solve this question, follow the steps below.

Step 01: Create an equation such that x equals the decimal number.

`x=0.\bar{210}`

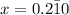
Let this equation be equation 01.

Step 02: Create a second equation by multiplying both sides of equation 01 by 10³.

We multiply both sides by 10³ since the decimal has 3 repeating numbers.

Since 10³ = 1000:

`1000x=210\bar{.210}`

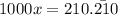
Let this equation be equation 02.

Step 03: Subtract equation 01 from equation 02.

`\begin{gathered} 1000x=210.210\ldots \\ -\text{ }x\text{ }=\text{ }0.210... \\ _(------------) \\ 1000x-x=210.210\ldots-0.210\ldots \\ 999x=210 \end{gathered}`

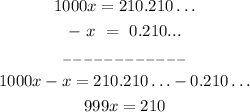
Step 04: Divide both sides of the equation by 999 to find x.

`\begin{gathered} (999)/(999)x=(210)/(999) \\ x=(210)/(999) \end{gathered}`

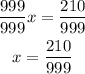
Simplify the fraction by dividing both the numerator and the denominator by 3.

`\begin{gathered} x=((210)/(3))/((999)/(3)) \\ x=(70)/(333) \end{gathered}`

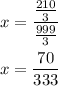
Since

`\begin{gathered} x=0.\bar{210} \\ \text{and} \\ x=(70)/(333) \\ \text{Then,} \\ 0.\bar{210}=(70)/(333) \end{gathered}`

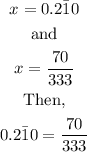
Answer:

`0.\bar{210}=(70)/(333)`