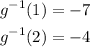Given: A one-to-one function g with the following values-
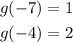
Required: To find the value of
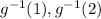
Explanation: The inverse of a function is obtained by interchanging the domain and the range of the function.
Hence the inverse of the function at x=1 and x=2 is
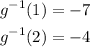
Final Answer: