Question

select the true statements that if true would prove triangle a CD is congruent to triangle BCD by hypotenuse leg. -205

Answer 1

eies,doekw0 2,e9e,e 02p hypotenuse led

Answer 2

Consider that both triangles, ADC and CDB are right triangles (otherwise proving congruence by using the HL postulate would make no sense).

Remember that, according to the HL (hypotenuse leg) postulate, if two right triangles have the same measure of their hypotenuse and the same measure of another correspondent side, both triangles are congruent.

Then, we need the hypotenuse to be the same length, this is

`AD\cong DB`

This is the condition that always has to be true.

Now, either of the correspondent remaining sides of the triangles can be the same measure, this is

`\begin{gathered} AC\cong CB \\ or \\ DC\cong DC \end{gathered}`

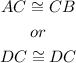
So, the options that are correct are:

`\begin{gathered} AC\cong BC\rightarrow leg \\ AD\cong BD\rightarrow Hypotenuse \\ AD\perp DC\to\text{right angle} \end{gathered}`

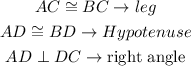
Notice that they share the side DC, so this could also be considered when applying the HL postulate and then AC=BC would be superfluous.

If we only can choose two options, we need to select:

`\begin{gathered} AD\cong BD\rightarrow Hypotenuse \\ AD\perp DC\to\text{right angle} \end{gathered}`