Step 1. We know the measure of all of the angles in the triangle and the measure of side b:
Required: Find the measure of sides a and c to the nearest tenth.
Step 2. To find a and c we use the law of sines.
A, B, and C are the angles of the triangle and a, b, and c are the sides. The law of sines is:

In our case, substituting the known values:

Step 3. Using the first equality we can find the value of a:
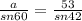
Solving for a:
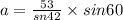
Solving the operations:

The result is already rounded to the nearest tenth.
Step 4. Using the second equality from step 2
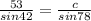
We will be able to find the value of c.
Solving for c:

Solving the operations:

The result is already rounded to the nearest tenth.
Answer:
