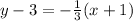Question

23. What is an equation in point-slope form for the line perpendicular to y = 3x - 11 that contains (-1, 3)?

Answer 1

For a line perpendicular to another on the coordinate grid, the slope of one would be a negative inverse of the other.

The equation of the line given here is;

`y=3x-11`

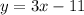
The slope of the line in this equation is the coefficient of x, that is, the slope is 3.

Therefore, this simply means the slope of the line perpendicular to this one would be;

`m=-(1)/(3)`

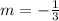
Note that this is the inverse of the slope m = 3, with a negative sign.

The equation of a line in point slope form is given as;

`y-y_1=m(x-x_1)`

Where the variables are;

`\begin{gathered} (x_1,y_1)=(-1,3) \\ m=-(1)/(3) \end{gathered}`

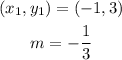
Substituting for the known values, we can now write out the equation in point-slope form as follows;

`\begin{gathered} y-y_1=m(x-x_1) \\ y-3=-(1)/(3)(x-\lbrack-1\rbrack) \\ y-3=-(1)/(3)(x+1) \end{gathered}`

ANSWER:

`y-3=-(1)/(3)(x+1)`