Question

The equation of a parabola is y = x(squared)+ 2x + 8. Write the equation in vertex form

Answer 1

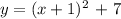
`y=(x+1)^2\text{ + 7}`Step-by-step explanation:
`\begin{gathered} \text{Given:} \\ y\text{ = }x^2\text{ + 2x + 8} \end{gathered}`

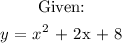
The equation of parabola in vertex form:

`y\text{ = a(x }-h)^2\text{ + k}`

where (h, k) is the vertex

`\begin{gathered} \text{h = }(-b)/(2a) \\ k\text{ = f(-b/2a)} \end{gathered}`

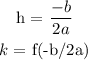
From the equation given: a = 1, b = 2, c = 8

`\begin{gathered} h\text{ = }(-2)/(2(1))\text{ = -2/2} \\ h\text{ = -1} \\ \\ \text{let f(x) = x}^2\text{ + 2x + 8} \\ k=f(-(b)/(2a))\text{ = f(-1)} \\ f(-1)=(-1)^2\text{ + 2(-1) + 8 = 1 - 2 + 8} \\ k\text{ = f(-1) = 7} \end{gathered}`
`\begin{gathered} \text{The equation substituting the vertex:} \\ y=a(x-(-1))^2\text{ + 7} \\ y=a(x+1)^2\text{ + 7} \end{gathered}`

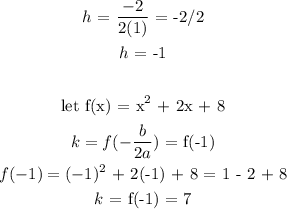
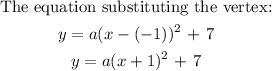
We need to find a. To get a, we will use the y-intercept.

The value of y when x = 0

`\begin{gathered} y\text{ = (0)}^2\text{ + 2(0) + 8} \\ y\text{ = 8} \\ \text{The y intercept: }(0,\text{ 8)} \end{gathered}`

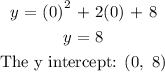
Substitute for x and y in the vertex equation using the y-intercept:

`\begin{gathered} y\text{ = a}(x+1)^2\text{ + 7} \\ x\text{ = 0, y = 8} \\ 8=a(0+1)^2\text{ + }7 \\ 8\text{ }=a(1)^2\text{ + 7} \\ 8\text{ = a + 7} \\ a\text{ = 8 - 7} \\ a\text{ = 1} \end{gathered}`

The equation in vertex form:

`\begin{gathered} y=1(x+1)^2\text{ + 7} \\ y=(x+1)^2\text{ + 7} \end{gathered}`