Step 1: Write out the formula and the special triangle
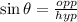

Step 2 (a): Write out the given values and substitute them into the formula
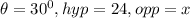
Therefore,

Step 2(b) Note that for the special triangle in Figure 1
The adjacent of angle 30 degrees is equal √3(the opposite of angle 30 degrees)
Therefore,
![y=\sqrt[]{3}x](https://img.qammunity.org/2023/formulas/mathematics/college/g9z7xeapl1uh9ro4rlfj3uv7jdlsuwu53l.png)