1 Answer

Kaysush · Answer 1 · 2023-04-27T06:27:05+0000

From the given values for the two side of triangles in the side of the present pyramid, we can do as follows:

We are going to use the Pythagorean theorem to find the height of this triangle.

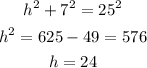
$\begin{gathered} h^2+7^2=25^2 \\ h^2=625-49=576 \\ h=24 \end{gathered}$

Now, we can use the values for the base and height of the triangle that is one of the sides of the pyramid to calculate its area:

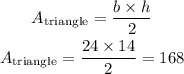
$\begin{gathered} A_{\text{triangle}}=(b* h)/(2) \\ A_{\text{triangle}}=(24*14)/(2)=168 \end{gathered}$

And because the pyramid has 8 sides with the same geometry and size, all we need now is multiply this value by 8.

$\begin{gathered} A_{\text{lateral}}=8* A_(triangle) \\ A_{\text{lateral}}=8*168 \\ A_{\text{lateral}}=1,344 \end{gathered}$

From this, we can say that the answer is 1,344

Please log in or register to add a comment.