Question

Given the function f(x) = 2(x + 1)^2 - 4Determine:(a)the vertex of parabola (b)x intercept (c)y intercept

Answer 1

(a)Vertex = (-1, -4)

(b)x-intercepts are -2.41 and 0.41

(c)y-intercept = -2.

Step-by-step explanation:

Given the function

`f\mleft(x\mright)=2\left(x+1\right)^2-4`

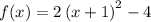
(a)Comparing with the vertex form of a quadratic function

`\begin{gathered} f(x)=a\left(x-h\right)^2+k \\ \text{where (h,k) is the vertex} \end{gathered}`

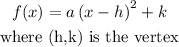
The vertex of the parabola is: (-1, -4)

(b)To determine the x-intercepts, we set f(x)=0 and solve for x.

`\begin{gathered} f(x)=2(x+1)^2-4=0 \\ 2(x+1)^2-4=0 \\ 2(x+1)^2=4 \\ (x+1)^2=(4)/(2) \\ x+1=\pm√(2) \\ x=-1\pm√(2) \\ x=-1-√(2)\lor x=-1+√(2) \\ x=-2.41\lor x=0.41 \end{gathered}`

The x-intercepts are -2.41 and 0.41.

(c)The y-intercept occurs when x=0.

Therefore:

`\begin{gathered} f(0)=2(0+1)^2-4 \\ =2-4 \\ =-2 \end{gathered}`

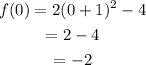
The y-intercept of the function is -2.