Question

Solve for k. 4k – 10 = 8 . = Stuck? Watch a video or use a hint.

Answer 1

For the given equation , we have to cross multiply the equation and equate like terms and unlike terms!

Refer attachment for working!

Answer 2

We are given the following equation

`(4k-10)/(k)=8`

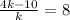
Let us solve this equation for k

Step 1:

Multiply both sides of the equation by k

`\begin{gathered} k\cdot((4k-10))/(k)=8\cdot k \\ 4k-10=8k \end{gathered}`

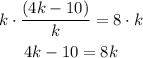
Step 2:

Subtract 8k from both sides of the equation

`\begin{gathered} 4k-8k-10=8k-8k \\ -4k-10=0 \end{gathered}`

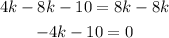
Step 3:

Add 10 to both sides of the equation

`\begin{gathered} -4k-10+10=10 \\ -4k=10 \end{gathered}`

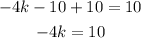
Step 4:

Finally, divide both sides of the equation by -4

`\begin{gathered} (-4k)/(-4)=(10)/(-4) \\ k=-(10)/(4) \\ k=-(5)/(2) \end{gathered}`

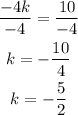
Therefore, the value of k is equal to -5/2