Given the original length is
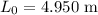
Given the initial temperature is

The final temperature is
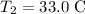
The linear thermal expansion is given by

Here, alpha is the coefficient of linear thermal expansion.
For concrete the value of the coefficient is

The formula is used to calculate the final height of a concrete.
Substitute the given values.
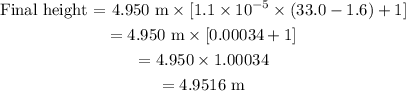
Thus, the value of the final height is 4.9516 m.