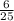Question

Consider a prismatic dice with sides 1, 2, 3, and 4. When rolled, P(1) = 1/10 , P(2) = 2/10 , P(3) = 3/10 , P(4) = 4/10What is the probability of getting an odd outcome on either of two rolls of the dice?

Answer 1

Given:

A prismatic dice with sides 1,2,3, and 4.

Where when rolled

`\begin{gathered} P(1)=(1)/(10) \\ P(2)=(2)/(10) \\ P(3)=(3)/(10) \\ P(4)=(4)/(10) \end{gathered}`

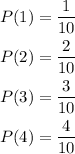
The probability of getting an odd outcome on either of two rolls of the dice is expressed as

`P(odd\text{ outcome on either of two rolls\rparen=P\lparen1 and 2\rparen or P\lparen1 and 4\rparen or P\lparen3 and 2\rparen or P\lparen3 and 4\rparen}`

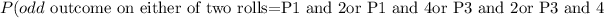
Thus, we have

`\begin{gathered} P(odd\text{ outcome on either of two rolls\rparen = \lparen}(1)/(10)*(2)/(10))+((1)/(10)*(4)/(10))+((3)/(10)*(2)/(10))+((3)/(10)*(4)/(10)) \\ =(1)/(50)+(1)/(25)+(3)/(50)+(3)/(25) \\ =(6)/(25) \end{gathered}`

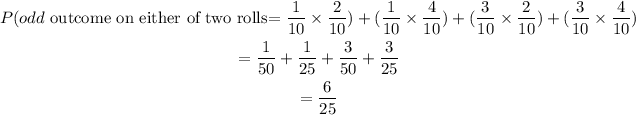
Hence, the probability of getting an odd outcome on either of two rolls of the dice is evaluated to be

`(6)/(25)`