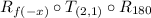Question

Reflect AABC over the y-axis, translate by (2, -1), and rotate the result 180° counterclockwise aboutthe origin. Plot AA'B'C'' on the grid below.

Answer 1

Here, we want to perform transformations operration on the line

We start by writing out the coordinates of the sides of the triangle

We have this as;

A (2,1)

B (2,-4)

C (4,-2)

The first thing we will do is to reflect over the y-axis

We have this as;

`(x,y)\text{ }\rightarrow\text{ (-x,y)}`

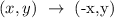
So, we have the initial coordinates as follows;

`\begin{gathered} A^(\prime)\text{ (-2,1)} \\ B^(\prime)\text{ (-2,-4)} \\ C^(\prime)\text{ (-4,-2)} \end{gathered}`

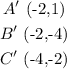
The next thing to do is to translate by the given coordinate. What this mean is that we add 2 to the x-axis value and subtract 1 from the y-axis value

We have this as;

`\begin{gathered} A^(\doubleprime)\text{ (-2 + 2 , 1-1) = A''(0,0)} \\ B^(\doubleprime)\text{ (-2 + 2, -1-4) = B''}(0,-5) \\ C^(\doubleprime)\text{ (-4+2, -2-1) = C'' (-2,-3)} \end{gathered}`

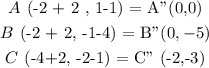
Lastly, what we have to do is to rotate the result countercloclwisely about the origin

The rule for this is;

`(x,y)\rightarrow(-x,-y)`

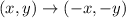
So, from the second transformation, we have;

`\begin{gathered} A^(\doubleprime)^(\prime)(0,0) \\ B^(\doubleprime)^(\prime)(0,5) \\ C^(\doubleprime)^(\prime)(2,3) \end{gathered}`

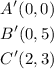
We then proceed to identfy these points on the plot and join so that we complete the triangle shape

The transformation rule is;

`R_(f(-x))\circ T_((2,1))\circ R_(180)`