For any polynomial of degree n, we can write the general form as:
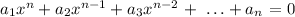
Where:

We are given that the degree of the polynomial is 5 and it has a leading coefficient of 7 and a constant term of 6.
Checking through the options:
Option A
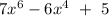
It has a degree of 6, hence it is wrong.
Option B
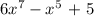
It has a leading coefficient of 6, hence it is wrong.
Option C

It has a leading coefficient of 6, hence it is wrong.
Option D
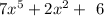
It has a degree of 5, a leading coefficient of 7, and a constant term of 6, hence it is correct
Answer: Option D