Since theta is the angle between the ladder and the ground
Since the length of the ladder is 16 feet
Since the height of the window from the ground is 12 ft 6 inches
We have to change the inches to feet
Since 1 foot = 12 inches, then
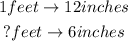
By using cross multiplication

That means 6 inches = 0.5 feet
The height of the window is 12.5 feet
By using the sine ratio
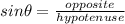
The opposite = 12.5
The hypotenuse = 16
Substitute them in the rule
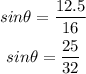
By using the inverse of sine
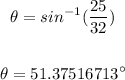
We can round it to 1 decimal place
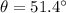
The answer is 51.4 degrees to the nearest one decimal place