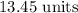Answer:
the side length x is;
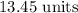
Step-by-step explanation:
Given the right triangle in the attached image.
To calculate the value of x, Let us apply the Pythagorean theorem;
![\begin{gathered} c^2=a^2+b^2 \\ c=\sqrt[]{a^2+b^2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/bqzsis30q1evi8wis8kvh1zcb4e2sbt38a.png)
From the given triangle;
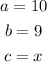
substituting the values;
![\begin{gathered} c=\sqrt[]{a^2+b^2} \\ x=\sqrt[]{10^2+9^2} \\ x=\sqrt[]{100+81} \\ x=\sqrt[]{181} \\ x=13.45 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/8hb4z55wj25e38vfsorueqrijro52q508g.png)
Therefore, the side length x is;