Lets suppose that m-1 is always greater than 1-m. Then, we can write:
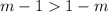
If we move -1 to the right hand side and -m to the left hand side, we get
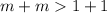
which gives
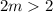
If we move the coefficient of m to the right hand side (2 is positive so it doesnt flip the inequality symbol) we have
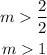
Therefore, Jon is correct if and only if m is greater than 1.