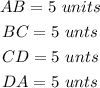Explanation
We are given the following coordinates:
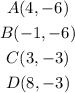
We are required to graph the coordinates given and find the distance of the following:
The points can be graphed as follows:
We know that the distance between two points can be calculated as:
Therefore, we can determine the distance of AB as:
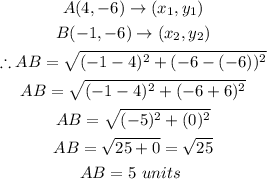
We can determine the distance of BC as:
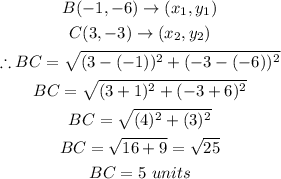
The value of CD is:
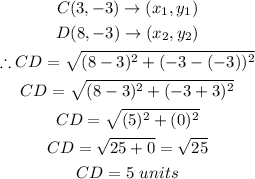
Finally, the value of DA is:
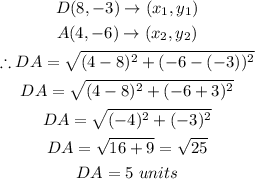
Hence, the answers are: