Question

A cloud has a potential difference relative to a car of 9.16 x 108 volts. During a lightning storm, a charge of 15.4 coulombs travels through this potential difference. If the energy released could somehow be harnessed and converted into kinetic energy, how fast could Mr. Baud's 2020 Kia Sorento move? His car has a mass of 1780 kilograms and starts from rest. Include units in your answer. Put the answer with 3 significant digits.

Answer 1

In order to find the energy stored by the car, let's use the formula below:

`E=Q\cdot V`

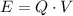
Where E is the electrical energy, Q is the charge and V is the potential difference (voltage).

So, for Q = 15.4 C and V = 9.16 * 10^8 V, we have:

`\begin{gathered} E=15.4\cdot9.16\cdot10^8 \\ E=141.064\cdot10^8\text{ J} \end{gathered}`

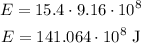
Now, converting this energy into kinetic energy, we have:

`\begin{gathered} E_k=(mv^2)/(2) \\ 141.064\cdot10^8=(1780\cdot v^2)/(2) \\ 141.064\cdot10^8=890v^2^{} \\ v^2=(141.064)/(890)\cdot10^8 \\ v=0.398119\cdot10^4 \\ v=3981.19\text{ m/s} \end{gathered}`

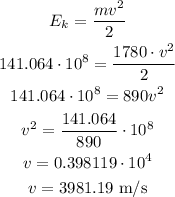
Therefore, putting the answer with 3 significant digits, the velocity of the car would be 3980 m/s.