Given:
vertex : (-5, -10)
Point : (0, 15)
A quadratic equation can be written in its vertex form as:
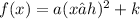
Where (h,k) is the coordinate of the vertex and a is the constant multiplier
For this problem,

Hence, we can write:
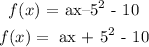
Since the point (0, 15) lies on the curve, we can substitute and solve for a:
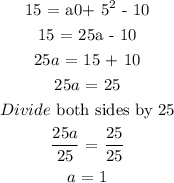
Hence, in vertex form, the quadratic equation is:
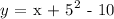
Answer: Option C