Question

An electrician leans an extension ladder against the outside wall of a house so that it reaches an electric box 34 feet up. The ladder makes an angle of 63° with the ground.Find the length of the ladder. Round your answer to the nearest tenth of a foot if necessary.

Answer 1

From the diagram above, the left side shows the ladder leaning on the wall. This has made a right-triangle as seen in the right side of the image. So we will solve for the length of the ladder given as h by using the trig-ratio SOHCAHTOA

The opposite side is 34 feet and the hypotenuse is h. So we will use SOH, we have

`\begin{gathered} SOHsin\theta=(opposite)/(hypotenuse) \\ sin63\degree=(34)/(h) \\ (sin63)h=34 \\ h=(34)/(sin63) \\ h=38.159092 \\ h=38.2\text{ feet} \end{gathered}`

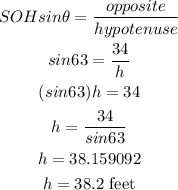
Hence the answer is 38.2 feet