Question

A manufacturer produces coffee makers. He estimates that by selling them for X dollars each, he will be able to sell 200-3X each week. Determine what price will yield a maximum revenue. Round your answer to two decimal places.

Answer 1

Price = x

Quantity = 200 - 3x

The formula for revenue is Price x Quantity. To be able to get the maximum revenue, let's multiply the price of each coffee maker and the number of coffee makers sold each week.

`\begin{gathered} R=P* Q \\ R=x(200-3x) \\ R=200x-3x^2 \end{gathered}`

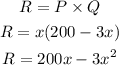
The formula for the revenue as we can see above is R = 200x - 3x². To be able to solve for the maximum revenue, let's solve for the vertex of this quadratic equation.

The formula for the vertex is:

`x=-(b)/(2a)`

where b = the first degree term and a = second degree term.

On our equation, our a = -3, and b = 200. Let's plug it in to our formula above.

`x=-(200)/(2(-3))=-(200)/(-6)=33.33`

Therefore, the price that will yield a maximum revenue is when it is $33.33.