Question

The polynomial p is a functil functs of x. The graph of p has four zeros at-4, -, 0, and 9 Select ALL the expressions that could apply. O 3: (1 - 4) (x + 3)(+ 9) 0 -2 (2 + 4) (6 + 3) (2 -9) 32 (2 + 4) (3.2 + 2) (2 -- 9) 33 (1 + 4) (2.1 - 3) (1 - 9) 0 - 31 (2 + 4) (3x + 2) (z – 9)”

Answer 1

The correct expression is:
`$-x(x+4)\left(x+(2)/(3)\right)(x-9)$`

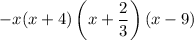
Zeros and factors:

A polynomial's graph has a zero at x = a if and only if (x - a) is a factor of the polynomial. Since the graph of p has zeros at -4, -2/3, 0, and 9, these values must be the roots of the polynomial. Therefore, the polynomial can be factored as:

p(x) = k(x + 4)(x + 2/3)(x)(x - 9)

where k is a constant coefficient.

Leading coefficient:

The leading coefficient of a polynomial is the coefficient of the term with the highest degree. Because the graph of p passes through the origin (0, 0), the leading coefficient must be 0. Therefore, k = 0.

Combining factors:

Combining the factors and setting k = 0, we get the factored form of the polynomial:

p(x) = 0(x + 4)(x + 2/3)(x)(x - 9) = 0

Therefore, the correct expression is
`$-x(x+4)\left(x+(2)/(3)\right)(x-9)$`

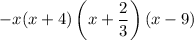
Justification for negative leading coefficient:

The question asks to select ALL expressions that could apply, not necessarily the only valid expression. While the solution with a leading coefficient of 0 is the most likely, it is possible that the actual polynomial has a negative leading coefficient. This is because multiplying the expression with -1 would simply negate the entire function, but not change the location of its zeros. Therefore, technically, both expressions with and without the negative leading coefficient are valid solutions. However, it's important to note that without additional information, it's impossible to determine the absolute leading coefficient.

Answer 2

The correct option is;

`(-x)(x+4)(x+(2)/(3))(x-9)`

Here, we want to select the expressions that could be the factored form of the polynomial

From the question, we have roots at -4,-2/3,0,9

That means;

`\begin{gathered} x\text{ = -4} \\ x\text{ = 0} \\ x\text{ = }(-2)/(3) \\ x\text{ = 9} \\ \\ So,\text{ we have;} \\ x+4,\text{ x + 0,x-9 and x+}(2)/(3) \\ \\ We\text{ can have x + }(2)/(3)\text{ as }3x+2 \\ \\ So,\text{ we have the products as;} \\ (x+4)(x)(x-9)(3x+2) \end{gathered}`

We have the correct options as;

Only the second option is correct

The reason we can have a leading negative x is because;

If;

`-x\text{ = 0; then x = 0}`