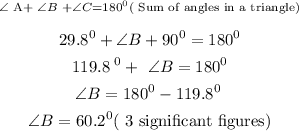SOLUTION:
Step 1:
In this question, we are given the following:
Step 2:
We need to get the values of B, b, and c.
Part A:
To get the value of b, we have to do the following:
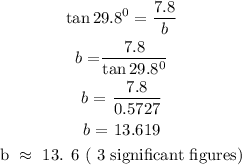
Part B:
To get the value of c, we need to do the following:
Using Pythagoras' theorem, we have that:
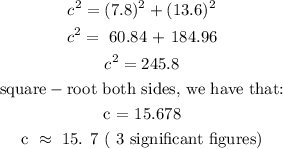
Part C:
To get the value of B, we need to do the following: